
|
No Form Action Theory |

|
No Form Action Theory |
 https://orcid.org/0009-0005-4318-2670
https://orcid.org/0009-0005-4318-2670
The previous exposition of no form action theory has laid the foundation for applying mathematics to philosophy. The relationships between the three no form actions already contain certain mathematical structures. In this section, we will extract these mathematical structures and then proceed with mathematical derivations to see what conclusions can be drawn. This section's approach to studying philosophy is completely different from before - it aims to use rigorous mathematical calculations to understand relationships between concepts and establish concepts based on mathematical structures. This makes philosophical research systematic, rigorous and precise like scientific research, elevating philosophy to its rightful position. This can address long-standing criticisms of philosophy as being too vague, subjective, and lacking clear progress. Previous traditional philosophical research only used imprecise speculation and vague intuition (although these are essential and their value cannot be denied, they do have limitations), resulting in philosophical views that were often unconvincing. Now these philosophical limitations will become history - with no form action theory successfully constructed on rigorous mathematical foundations, philosophical research will rise to a new level. Philosophy will enter a new era, transforming from an ancient field of study into a mature discipline.
Since this section primarily uses group theory from mathematics, I'll first briefly introduce groups for readers who are unfamiliar with them.
Let G be a non-empty set. If we define a binary operation "·" on G that satisfies the following conditions:
(1) Closure: For any a,b in G, there exists a unique c such that a·b=c;
(2) Associativity: For any a,b,c in G, (a·b)·c=a·(b·c).
(3) Identity element: There exists an element e in G such that e·a=a·e=a.
(4) Inverse element: For any a in G, there exists b in G such that a·b=b·a=e. When a has an inverse, b is called the inverse element of a, denoted as a-1.
Then G is called a group.
1) The Klein four-group provides a structure that can represent the three actions of no form action theory and their mutual transformations. The Klein four-group was chosen because it captures the core idea of cyclic transformation between different elements, which aligns well with the concept of mutual transformation among the three actions.
I. Klein Four-Group Structure:
The Klein four-group, typically denoted as V or K4, has four elements {e, a, b, c}, with the following operation table:
· e a b c
e e a b c
a a e c b
b b c e a
c c b a e
II. Key Properties of Klein Four-Group:
Each element is its own inverse: a + a = e, b + b = e, c + c = e
Operations are commutative: a + b = b + a = c, etc.
Mapping No Form Actions to Group Elements:
e: represents no form, emphasizing that no form has not yet combined with form and will not produce any no form action. This serves as the identity element.
a: represents manifestation action
b: represents motive force action
c: represents isolation action
III. Explaining Group Operations:
The group operation (+) represents one no form action transforming into a third no form action through another no form action.
(1) x + e = x: No form e as the identity element does not change any action.
(2) c + c = e: According to no form united transformation, isolation needs another type of no form action to transform into a third no form action. Therefore, isolation action cannot change on its own, which means nothing actually happens. Isolation action acting upon itself produces no effect, belonging to pure no form. Thus, c + c results in e.
(3) b + b = e: Following the same logic, b + b results in e.
(4) a + a = e: Following the same logic, a + a results in e.
(5) a + b = c: Represents that manifestation transforms into isolation through motive force.
(6) a + c = b: Represents that manifestation transforms into motive force through isolation.
(7) b + c = a: Represents that motive force transforms into manifestation through isolation.
(8) b + a = c: Represents that motive force transforms into isolation through manifestation.
(9) c + a = b: Represents that isolation transforms into motive force through manifestation.
(10) c + b = a: Represents that isolation transforms into manifestation through motive force.
Some things' transformations between manifestation, motive force, and isolation are commutative (e.g., a+b=b+a=c), which can be represented by this group. For example, the three basic laws of formal logic work this way - any two laws can transform into the third regardless of order. Clearly, if A (manifestation), B (motive force), C (isolation) constitute a no form integrated transformation, they can be represented by this group. Conversely, if A (manifestation), B (motive force), C (isolation) form such a group, then they constitute a no form integrated transformation. This shows that this group is meaningful - it represents no form integrated transformation.
Thus, we call this group No Form V. No Form V elevates no form integrated transformation from a philosophical concept to a mathematical level, making it more precise and universal. No Form V is the mathematical abstract expression of no form integrated transformation. It can not only represent no form integrated transformation but also verify whether a transformation meets the requirements of integrated transformation, and vice versa. No form integrated transformation is no longer just a philosophical idea but a mathematical model that can be operated and verified. This means it can actively operate on philosophical concepts rather than just abstractly describe them. This gives no form action theory greater theoretical rigor and universality.
However, more importantly for this No Form V that I constructed, the Klein four-group is isomorphic to Z2×Z2. Z2 (or C2) = {0,1}, Z2×Z2 = {(0, 0), (0, 1), (1, 0), (1, 1)}, and operations in Z2×Z2 are componentwise modulo 2 addition: (x1, y1) + (x2, y2) = (x1 + x2 mod 2, y1 + y2 mod 2). If we use 0 to represent no form (note that 0 here emphasizes the relationship with form) and 1 to represent form, then Z2 can be viewed as a cyclic group composed of no form and form. The operation in cyclic group Z2 is modulo 2 addition: {0, 1}, with 0 as the identity element, 0 + 0 = 0 (mod 2), 0 + 1 = 1 (mod 2), 1 + 0 = 1 (mod 2), 1 + 1 = 0 (mod 2). The equation 1 + 1 = 0 (mod 2) can be interpreted as form cannot develop from itself - form can only develop through no form (for example, 0 + 1 = 1 (mod 2)), it can only develop by returning to no form, hence 1 + 1 = 0; conversely, no form also needs form to function. In other words, this demonstrates their indivisibility. Therefore, Z2 represents the relationship between no form and form.
Since Z2 contains the relationship between form and no form, No Form V can be seen as derived from the direct product Z2×Z2. Thus, (0, 0) can be viewed as the identity element no form e (although no form changes from 0 to e remains no form, its identity changes: from a relationship with form to a relationship with three no form actions), (0, 1) can be viewed as manifestation action a, (1, 0) can be viewed as motive force action b, and (1, 1) can be viewed as isolation action c. In other words, the elements 0 (representing no form) and 1 (representing form) in Z2 combine to form three concrete no form actions in No Form V. From (0, 0)... to (1, 1), this combination process indicates a gradual strengthening of form: (0, 0) represents no no form action, (0, 1) represents manifestation action a where no form dominates and form is secondary, (1, 0) represents motive force action b where form dominates and no form is secondary, (1, 1) represents isolation action c where form completely dominates. This process can be seen as a dynamic demonstration of the interaction and gradual transformation between no form and form, while also revealing the generation order and interrelationships of different actions in no form theory. No Form V can be viewed as an extension of Z2, where the relationship between form and no form is not just a logical complementary relationship, but achieves dynamic transformation and balance through manifestation action, motive force action, and isolation action.
Since Z2 is the only form for a group with two elements, and the product of two Z2 can only be a direct product, the group expanded from Z2 itself (which has the relationship between form and no form) can only be No Form V (in terms of isomorphism). This means that from a mathematical perspective, we have also determined that there are only three fundamental actions: manifestation, motive force, and isolation, and these three actions are by no means arbitrary. In fact, the creation process of no form action theory started from a two-dimensional theory composed of form and no form, then proceeded to the three actions of no form, which follows the same order as expanding from Z2 to No Form V. However, the creation process of no form action theory was only conducted through intuitive analysis, without clearly establishing the relationship between the two-dimensional theory and the three actions of no form. Through mathematical methods, we have clearly obtained their relationship: the three actions of no form can be obtained simply by different combinations of form and no form. In fact, it would be very difficult to conceive this point using only intuitive analysis. But this also indicates that the no form action theory created through intuition is not arbitrary, but was implicitly guided by potential logical and mathematical structures.
2) Direct Product: No Form V×V
We can also create a direct product group from (No Form V) × (No Form V), called: No Form V×V. With No Form V1 = {e,a,b,c} and No Form V2 = {e,a,b,c}, No Form V×V = {(e,e),(e,a),(e,b),(e,c), (a,e),(a,a),(a,b),(a,c), (b,e),(b,a),(b,b),(b,c), (c,e),(c,a),(c,b),(c,c)}. Thus, No Form V×V has 16 elements with two dimensions: V1 and V2. Since No Form V×V is a group, its elements can be operated on: (x,y)+(m,n)=(x+m,y+n), for example, (a,c)+(e,b)=(a+e,c+b)=(a,a). This means that their operations occur within their respective dimensions, with operations in the two dimensions being independent of each other. This direct product actually transforms one-dimensional no form united transformation into two-dimensional no form united transformation. No Form V×V has 16 elements, so there are 16×16=256 operations between these 16 elements, and since it is a group, these 256 operations still yield 16 operational results.
The product between groups is also a type of operation, but it is already different from operations between elements within a single group. This type of operation between groups has become a form like (x,y), where x belongs to No Form V1 and y belongs to No Form V2. What philosophical implications does this have?
From the perspective of one no form action (x) viewing another no form action (y) means x in y. For example, viewing isolation action from the perspective of motive force action means independence, which is motive force action within isolation action. If we view this as an operation, for example, viewing isolation action (c) from motive force action (b) perspective would be: (c,b). We can call this type of operation a "perspective operation." Thus, the product operation between groups is a "perspective operation." This operation breaks through single-dimensional limitations and demonstrates multi-dimensional connections between no form actions. "Perspective operation" emphasizes the importance of viewpoint, meaning that an action may have different meanings under different perspectives. For example, "independence" and "generation" are two different manifestations under mutual perspectives.
The 16 elements of No Form V×V represent these concepts respectively: no form (e,e), self (a,a), being-for-itself (b,b), self-limitation (c,c), transparency (e,a), freedom (e,b), being (e,c), manifestation (a,e), motive force (b,e), isolation (c,e), immediacy (a,b), identity (a,c), generation (b,c), change (b,a), independence (c,b), distinction (c,a).
Since these 256 operations are quite extensive, we will study their relationships by categorizing and arranging the 16 elements of No Form V×V, as shown in the table below:
no form(e,e) manifestation (a,e) motive force(b,e) isolation (c,e)
self (a,a) transparency (e,a) freedom (e,b) being (e,c)
being-for-itself(b,b) change (b,a) generation (b,c) identity (a,c)
self-limitation(c,c) immediacy (a,b) independence (c,b) distinction (c,a)
This allows us to combine perspective operations with No Form V group operations, integrating different perspectives with no form united transformation. This enables complex philosophical problems to be expressed within a unified framework. No Form V is the basic structure of three no form actions, while No Form V×V is a higher-dimensional extension of this structure.
Elements in No Form V×V can undergo group operations. For example, identity(a,c) + independence(c,b) = change(b,a), which means identity and independence can transform into change, or that identity needs independence to transform into change. Furthermore, we can verify that these three form a no form integrated transformation, meaning they can transform into each other. This actually transforms one-dimensional no form integrated transformation into two-dimensional no form integrated transformation. Thus, we can obtain relationships between these concepts through group operations, a method that transcends pure description or intuitive assertion - in other words, relationships between concepts can be independently verified through mathematical operations. Compared to one-dimensional no form integrated transformation, the two-dimensional structure allows more concept interactions to be embedded in a more complex mathematical framework, making analysis deeper and more systematic. This is using mathematical operations to obtain relationships between concepts. In this way, we can discover previously undiscovered relationships between concepts. This two-dimensional group structure allows verification of whether three concepts satisfy the conditions of no form integrated transformation, namely that each concept's transformation into another concept requires the third concept. The introduction of group operations develops no form action theory from pure philosophical theory into a mathematical logical framework that can be operated and derived. It is not just a descriptive tool, but also an analytical and reasoning tool. This is a method that uses computational power to analyze and understand complex philosophical problems in previously impossible ways.
We can obtain all two-dimensional no form integrated transformations in No Form V×V, with a total of 35 groups, where the three concepts in each group can transform into each other:
(1) {transparency(e,a), freedom(e,b), being(e,c)}
(2) {transparency(e,a), manifestation(a,e), self(a,a)}
(3) {transparency(e,a), immediacy(a,b), identity(a,c)}
(4) {transparency(e,a), motive force(b,e), change(b,a)}
(5) {transparency(e,a), being-for-itself(b,b), generation(b,c)}
(6) {transparency(e,a), isolation(c,e), distinction(c,a)}
(7) {transparency(e,a), independence(c,b), self-limitation(c,c)}
(8) {freedom(e,b), manifestation(a,e), immediacy(a,b)}
(9) {freedom(e,b), self(a,a), identity(a,c)}
(10) {freedom(e,b), motive force(b,e), being-for-itself(b,b)}
(11) {freedom(e,b), change(b,a), generation(b,c)}
(12) {freedom(e,b), isolation(c,e), independence(c,b)}
(13) {freedom(e,b), distinction(c,a), self-limitation(c,c)}
(14) {being(e,c), manifestation(a,e), identity(a,c)}
(15) {being(e,c), self(a,a), immediacy(a,b)}
(16) {being(e,c), motive force(b,e), generation(b,c)}
(17) {being(e,c), change(b,a), being-for-itself(b,b)}
(18) {being(e,c), isolation(c,e), self-limitation(c,c)}
(19) {being(e,c), distinction(c,a), independence(c,b)}
(20) {manifestation(a,e), motive force(b,e), isolation(c,e)}
(21) {manifestation(a,e), change(b,a), distinction(c,a)}
(22) {manifestation(a,e), being-for-itself(b,b), independence(c,b)}
(23) {manifestation(a,e), generation(b,c), self-limitation(c,c)}
(24) {self(a,a), motive force(b,e), distinction(c,a)}
(25) {self(a,a), change(b,a), isolation(c,e)}
(26) {self(a,a), being-for-itself(b,b), self-limitation(c,c)}
(27) {self(a,a), generation(b,c), independence(c,b)}
(28) {immediacy(a,b), motive force(b,e), independence(c,b)}
(29) {immediacy(a,b), change(b,a), self-limitation(c,c)}
(30) {immediacy(a,b), being-for-itself(b,b), isolation(c,e)}
(31) {immediacy(a,b), generation(b,c), distinction(c,a)}
(32) {identity(a,c), motive force(b,e), self-limitation(c,c)}
(33) {identity(a,c), change(b,a), independence(c,b)}
(34) {identity(a,c), being-for-itself(b,b), distinction(c,a)}
(35) {identity(a,c), generation(b,c), isolation(c,e)}
Additionally, each triple plus the identity element (e,e) forms a fourth-order subgroup of No Form V×V, and they are all Klein four-groups. Since No Form V is a Klein four-group, it's interesting that No Form V×V, as an extension of No Form V, contains subgroups that are also Klein four-groups. This subtle recursion will be very useful.
There are also 16 two-dimensional no form integrated transformations of the form {no form(e,e), (x,y), (x,y)}. These are actually second-order subgroups of No Form V×V.
No Form V×V has 15 eighth-order subgroups, all isomorphic to the direct product Z2×Z2×Z2. Each subgroup is a closed system.
H1 = {no form(e,e), self(a,a), immediacy(a,b), identity(a,c), manifestation(a,e), transparency(e,a), freedom(e,b), being(e,c)}
H2 = {change(b,a), no form(e,e), being-for-itself(b,b), generation(b,c), motive force(b,e), transparency(e,a), freedom(e,b), being(e,c)}
H3 = {change(b,a), no form(e,e), self(a,a), isolation(c,e), motive force(b,e), manifestation(a,e), transparency(e,a), distinction(c,a)}
H4 = {no form(e,e), being-for-itself(b,b), immediacy(a,b), generation(b,c), identity(a,c), isolation(c,e), transparency(e,a), distinction(c,a)}
H5 = {independence(c,b), no form(e,e), being-for-itself(b,b), immediacy(a,b), isolation(c,e), motive force(b,e), manifestation(a,e), freedom(e,b)}
H6 = {change(b,a), independence(c,b), no form(e,e), self(a,a), generation(b,c), identity(a,c), isolation(c,e), freedom(e,b)}
H7 = {change(b,a), independence(c,b), no form(e,e), being-for-itself(b,b), identity(a,c), manifestation(a,e), being(e,c), distinction(c,a)}
H8 = {independence(c,b), no form(e,e), self(a,a), immediacy(a,b), generation(b,c), motive force(b,e), being(e,c), distinction(c,a)}
H9 = {change(b,a), no form(e,e), self(a,a), being-for-itself(b,b), self-limitation(c,c), immediacy(a,b), isolation(c,e), being(e,c)}
H10 = {no form(e,e), self-limitation(c,c), generation(b,c), identity(a,c), isolation(c,e), motive force(b,e), manifestation(a,e), being(e,c)}
H11 = {no form(e,e), self(a,a), being-for-itself(b,b), self-limitation(c,c), identity(a,c), motive force(b,e), freedom(e,b), distinction(c,a)}
H12 = {change(b,a), no form(e,e), self-limitation(c,c), immediacy(a,b), generation(b,c), manifestation(a,e), freedom(e,b), distinction(c,a)}
H13 = {change(b,a), independence(c,b), no form(e,e), self-limitation(c,c), immediacy(a,b), identity(a,c), motive force(b,e), transparency(e,a)}
H14 = {independence(c,b), no form(e,e), self(a,a), being-for-itself(b,b), self-limitation(c,c), generation(b,c), manifestation(a,e), transparency(e,a)}
H15 = {independence(c,b), no form(e,e), self-limitation(c,c), isolation(c,e), transparency(e,a), freedom(e,b), being(e,c), distinction(c,a)}
In No Form V×V, we discovered many oppositions, among which generation(b,c) and independence(c,b) are opposites (their opposition can be seen from the direction of elements (b,c) and (c,b)), and they are unified in cause; change(b,a) and immediacy(a,b) are opposites, unified in openness; identity(a,c) and distinction(c,a) are opposites, unified in ground. We have already seen these three pairs of opposites in the section "Dialectical Logic." However, these oppositions and unifications have so far only been obtained through conceptual analysis, not through mathematical operations, which will be used later to obtain these relationships.
We also see that: manifestation(a,e) and transparency(e,a), motive force(b,e) and freedom(e,b), isolation(c,e) and being(e,c) are all opposites. Note that this two-dimensional expression of these six concepts is an expression in the limit mode - for example, being(e,c) is the limit expression of "being," meaning viewing no form from the perspective of form, reaching being in a limit way. They are respectively the limits of these six concepts: essence and openness, subject and cause, substance and ground (as discussed in the section "Viewing No Form from the Perspective of Form"). This mode of expression emphasizes the role of limits.
What is essential is not open, what is open becomes phenomenon, so they are opposites. Similarly, if there is a subject, there should not be a cause, so they are opposites. Likewise, if there is an independently existing substance, there should not be a ground, so they are opposites. Through their opposition, we can also obtain the opposition of their limits. For example, substance and ground are opposites, their limits are respectively isolation(c,e) and being(e,c), and these two limits are also opposites, because isolation(c,e) can be seen as a limit-form substance, and being(e,c) can be seen as a limit-form ground (since viewing no form directly from form in an isolating way is "ground," the limit of continuously reducing the form of ground is being(e,c), being(e,c) is its own ground, and isolation(c,e) has a similar relationship with substance).
Ground and concrete are the same type of concept, but differences arise due to their relativity. For example, with concepts a, b, and c, if c is the ground of b, then b is the concrete of c, but b is also the ground of a, so b can be both concrete and ground, with differences arising only due to relativity. The direction of this relativity is opposite, so ground and concrete are opposites. By the same logic, cause and effect are opposites. Similarly, openness and concealment are opposites.
3) No Form V Extension Field
Using isolation action c as the identity element, we can extend this No Form V into field F4. As shown in the table below:
· e c a b
e e e e e
c e c a b
a e a b c
b e b c a
No Form V={e,a,b,c} extended to field F4: e as zero element, c as identity element, multiplication "·" as second operation.
We see that the three no form actions have been successfully constructed on the Klein four-group, and this group has been extended into field F4, which we can call "VFc (F represents field, c represents this field has c as identity element)." In this way, elements in VFc={e,a,b,c} can perform both "+" operations and "·" operations.
VFc as an extension field of No Form V={e,a,b,c} forms a group C3={c, a, b}, which is a cyclic group with identity element c, where the operations are: c·c=c, c·a=a, c·b=b, a·c=a, a·a=b, a·b=c, b·c=b, b·a=c, b·b=a.
Due to the inevitable reliability of mathematics, this C3 must have practical value. I explain this cyclic group as follows: it can be seen as a pure isolation world composed of isolation, where in the isolation world, motive force and manifestation can "ignore" isolation action. Isolation action acts like the background of the isolation world, where in the isolation background, we can focus on the interaction between motive force b and manifestation a, because isolation c provides a stable framework without needing to explicitly participate in the specific transformations between motive force and manifestation. This "ignoring" actually reflects the background role of isolation in no form action theory - it's not truly absent, but serves as an implicit condition for all transformations. Thus we can treat isolation c as the field's identity element. And field VFc serves as an intermediate transition between No Form V as the real world and C3 as the isolation world.
We see that element c as isolation action has become inert in C3, no longer interacting with other elements, with only interactions between b and a. This is because in the isolation world, motive force b and manifestation a are simulated by isolation action, with the real motive force and manifestation working behind them. In other words, in the isolation world, only motive force b and manifestation a are active, their interactions form isolation (a·b=c and b·a=c), which still conforms to no form united transformation.
C3 can be seen as a "projection" of No Form V onto elements b and a. Because in C3, element c becomes "inert," no longer interacting with other elements, just like a three-dimensional object being projected onto the x and y axes. Of course, these two types of projections are different, just similar. By "projecting" certain no form actions or dimensions, we can create simplified models or worlds that allow us to focus on and analyze specific aspects of reality in isolation, then reintegrate them into a more comprehensive understanding. This is a powerful method for philosophical exploration of complexity.
However, how should we understand the operations in C3? Let's first review isolation dialectical logic. A and non-A are opposites, A transforms into its opposite non-A through negation, A and non-A are opposites, and this opposition and unity is also a kind of opposition, therefore, through the opposition between A and non-A, the opposite "unity" is formed. Let's clarify affirmation and negation: "is" itself is manifestation, but it relates to the motive force action "affirmation." Similarly, "is not" is also manifestation, but it relates to the motive force action "negation." When we affirm a concept, we are using the manifestation "is" to transform into an isolated concept, this is no form united transformation, for example, a is b. In fact, affirmation and "is" are preconditions for isolated concepts, affirmation and isolated concepts are preconditions for "is," "is" and isolated concepts are preconditions for affirmation - they constitute no form integrated transformation. The same applies to "is not." "Is" and "is not" are opposites, affirmation and negation are also opposites. "Is" can transform into "is not" through negation, "is" can also transform into affirmation through "isolated concepts" - these are different no form united transformations.
Actually, two affirmations resulting in affirmation is only true in formal logic - in dialectical logic it's not like this. Negating A, then negating "the opposition between A and non-A," finally results in unity as affirmation. However, before negating A, A was first affirmed, and after negating A, non-A was affirmed. Therefore, we can say: affirming A, then affirming non-A, brings about "the opposition between A and non-A," thus leading to the negation of this opposition. This is actually saying: the result of two affirmations is negation. This is like in C3 where a·a=b (affirmation·affirmation=negation), b·b=a (negation·negation=affirmation). And a·b=c (or b·a=c) indicates that after affirmation and negation, things return to an isolation state without opposition (a state without affirmation and negation), this isolation state is the unified state of the opposition between A and non-A. Therefore, we can interpret c as an isolation state without manifestation and motive force, meaning c is inert, embodying the balance after the unity of opposites. Thus, C3 should be the mathematical structure we're looking for that can express dialectical logic - C3's operation mode naturally conforms to the isolation dialectical logic model.
Z2 only has basic symmetrical operations (such as simple inversion of 0 to 1), while No Form V expanded operations by introducing three no form actions (manifestation, motive force, isolation). C3 further provides a mathematical structure that can express isolation dialectical logic, comprehensively expressing the dynamic relationships of opposition, negation, and unity. This mathematical structure of dialectical logic actually expresses dialectical logic more completely, not only the negation of negation: negation·negation=affirmation, but also the affirmation of affirmation: affirmation·affirmation=negation. Affirmation and negation are always bound together, indivisible. This is actually a special manifestation of the indivisible identity of the three no form actions - since c as isolation has become inert, manifestation and motive force (affirmation and negation) have become indivisible. This indicates that dialectical logic is fundamentally about the tension and interaction between manifestation and motive force (affirmation and negation), rather than their simple resolution or cancellation. This reveals the deep structure of dialectical logic, making it no longer merely philosophical speculation, but a logical system with mathematical foundations.
Let's explain the operations in C3:
(1) x·c=x or c·x=x
Since c is inert, these two formulas don't change x.
(2) a·a=b
affirmation·affirmation=negation.
(3) b·b=a
negation·negation=affirmation.
(4) x·e=e (or e·x=e)
This formula shows that any multiplication between a no form action and no form action e is no form action e. This formula can be written as x·e=x·(x+x), for example, b·e=b·(b+b)=b·b+b·b=a+a=e, from this perspective we can understand why this formula results in e. This e serves as the identity element e in No Form V, and when No Form V is extended to field VFc, e participating in VFc's multiplication operation (x·e=e) becomes "nothingness" in the isolation world - from the perspective of the isolation world, this e is completely devoid of anything. At this point, this "nothingness" expresses that a concept has no attributes, or lacks a certain attribute, for example, a line segment has no area. This "nothingness" is a transformed no form, and is also a simulated "no form" in the isolation world. The significance of x·e=e is: all forms, when encountering "nothingness," are reduced to a state of complete absence of attributes.
We see that among the above formulas, only a·b=c and b·a=c are no form united transformations, while others are not. Furthermore, a, b, and c are no longer no form integrated transformations.
The above explanations of each operation show no contradictions and are self-consistent. These explanations actually represent the operational process of dialectical logic: affirmation and negation interact and transform into each other. The above structural analysis shows that the existence of a pure isolation world is a necessary phenomenon.
Since C3 is a cyclic group, it can be extended to dihedral group D3={1,a,a²,f,fa,fa²}. Here f is the reflection of C3. Then, set FA={f,fa,fa²} is the mirror image of C3, meaning FA and C3 are in opposite directions. Corresponding to C3's elements, D3 can be written as: D3={c,a,b,f,fa,fb} (these two notations are isomorphic), c is the identity element, and C3's mirror image is FA={f,fa,fb}. Thus, fa is FA's affirmation, and fb is FA's negation. For "negation b," what is seen in mirror image FA is affirmation fa. In other words, what is seen as negation in C3 is seen as affirmation in the mirror image, and vice versa. Similarly, for "affirmation a," what is seen in mirror image FA is negation fb. That is, what is seen as affirmation in C3 is seen as negation in the mirror image, and vice versa.
Therefore, for no form action theory, we can treat C3 as A and mirror image FA as non-A. Thus, since a·f=fb, f's action on a transforms the affirmation of A into the negation of non-A; similarly, b·f=fa transforms the negation of A into the affirmation of non-A. Conversely, fa·f=b, f's action on fa transforms the affirmation of non-A into the negation of A; similarly, fb·f=a transforms the negation of non-A into the affirmation of A.
The function of reflection f is isolation action. f makes A and non-A become isolated things. And f (as isolation) enables a (as manifestation) to transform into fb (as motive force), which is no form united transformation. Similarly, f (as isolation) enables b (as motive force) to transform into fa (as manifestation), which is also no form united transformation. This isolation action of f is already different from c (as isolation) - f is an isolation action at a higher level transcending c, because it acts on both opposing sides of A and non-A. Due to the action of f in D3, the relationship between A and non-A appears, which is a mathematical evolutionary extension, while C3 only remains at the level of operations on A.
(Note: f acts as isolation action, why not motive force action? Actually it could be, just that different perspectives lead to different functions. If we take a as manifestation and fb as motive force, then f is isolation action, but a can also be viewed as an independent thing, in which case it is an isolated thing, and its opposite fb would be its reverse manifestation, making f's action a motive force action with a negating function.)
We see that C3 expresses the relationship between affirmation and negation; while D3 expresses the relationship between A and non-A, their relationship being expressed through affirmation and negation. The analysis through C3 and D3 shows that the relationship between A and non-A is bound together with the relationship between affirmation and negation. They are both mutually opposed and unified together. Does this express the rules of formal logic?
Note that here we only have A and non-A, not yet the relationship between A and B. Therefore, according to no form action theory, affirming A is "A is A". Thus, f's action on a (a·f=fb) transforms "A is A" into "A is not non-A"; f's action on fb (fb·f=a) transforms "A is not non-A" into "A is A". Then, the law of contradiction is: A is not non-A. The law of excluded middle is: If A is A then it is not non-A, if A is not non-A then it is A. And the law of identity is: A is A.
We call these three basic laws of formal logic derived from D3 the "D3 version of the three basic laws of formal logic."
C3's operations are interpreted as "affirmation" and "negation," becoming the mathematical representation of dialectical logic itself, focusing on the dynamic interaction between affirmation and negation. D3, through operator 'f' and its transformations, becomes the mathematical representation of the relationship between A and non-A (the core of formal logic), but crucially, this relationship is expressed and understood through the dialectical dynamics (C3) of affirmation and negation. This suggests that formal logic, in its essence, is based on and derived from dialectical logic, as revealed by the mathematical structures of C3 and D3 in "no form action theory." Why express it as "A is A" and "A is not non-A"? These two expressions are manifestations of the two opposing aspects of "independence" as a characteristic of isolation: affirming self and negating non-self (as discussed in the section "Dialectical Logic"). And the dihedral group D3 perfectly fits this expression. Of course, we can also use "non-A is non-A" as the basis to evolve from non-A's perspective a non-A version of the three basic laws, and these two forms of logical laws are symmetrical.
From this, it's easy to see that the D3 version of the three basic laws constitutes a no form integrated transformation. The law of identity ("A is A") and the law of contradiction ("A is not non-A") together form the law of excluded middle: if A is A then it is not non-A, if A is not non-A then it is A. The law of identity ("A is A") and the law of excluded middle together yield the law of contradiction: from "A is A" and "if A is A then it is not non-A" we can obtain "A is not non-A". Similarly, the law of contradiction ("A is not non-A") and the law of excluded middle together yield the law of identity: from "A is not non-A" and "if A is not non-A then it is A" we can obtain "A is A".
We can define truth and falsity: let "A is A" be called true, and "A is not A" be called false. Thus truth and falsity become mutually exclusive relationships.
Since truth and falsity are mutually exclusive, we can substitute A=true and non-A=false into the D3 version of the three basic laws, which gives us the truth-false version of the three basic laws. Law of identity: truth is truth; law of contradiction: truth is not false; law of excluded middle: if truth is truth then it is not false, if truth is not false then it is truth.
Due to symmetry, we can also substitute A=false and non-A=true into the D3 version of the three basic laws, which gives us the false-truth version of the three basic laws. Law of identity: false is false; law of contradiction: false is not true; law of excluded middle: if false is false then it is not true, if false is not true then it is false.
For the expression "A is B", if A is indeed in set B, it can be written as an extension of "A is A" (as discussed in the "Formal Logic" section): "A is A in B" = true, abbreviated as "A is B" = true. Conversely, if A is not in set B, it can be written as an extension of "A is not A": "A is not A in B" = false, abbreviated as "A is B" = false. The extended form of "A is A" means we don't care about how specifically A is itself, but only focus on whether A is itself or not.
Thus, for the expression "A is B", regardless of its state, if we jointly use the true-false and false-true versions of the three basic laws, we can obtain:
(1) "A is B" is either false or true, and if not true then false. This can be simplified to: "A is B" must be either true or false. This is the expression of the law of excluded middle in traditional formal logic.
(2) "A is B" if true is not false, if false is not true. This can be simplified to: "A is B" cannot be both true and false. This is the expression of the law of contradiction in traditional formal logic.
(3) "A is A" is a universal expression.
These are the three basic laws of traditional formal logic.
It can be fully deduced that the three basic laws of traditional formal logic also constitute a no form integrated transformation.
Thus, using no form action theory and mathematical methods, and through the transition of "true-false" and "false-true" versions of the three basic laws, we evolved the three basic laws of traditional formal logic. Note that we are not proving the three basic laws of formal logic, but rather evolving the basic laws of formal logic that we commonly recognize from the most fundamental laws implied by Z2, which represents form and no form. Through the evolutionary process carried out by humans with dynamic thinking, these laws gradually manifested themselves. This indicates that the three basic laws of formal logic are not arbitrary or conventional, but originate from the underlying structure of the universe. These basic laws are necessary and inevitable. Through structured methods, this has been demonstrated step by step, and this demonstration process allows us to clearly see this necessity.
In the isolation world, "is" represents manifestation, while "negation" (associated with "is not") represents motive force. From the perspective of no form action theory, they are different no form actions that can only transform into each other but cannot substitute for each other, thus having mutual exclusivity. Their mutual exclusivity is what early traditional formal logic stated: something cannot both be and not be (Law of Contradiction); something must either be or not be (Law of Excluded Middle) - these are the formal logical laws of "is". Because "is" and "is not" are the most basic no form actions (in the isolation world), and the formal logical laws of "is" are the most abstract (meanwhile, our linguistic expression can only be based on "is"), we can only evolve concrete logical laws based on them, thus concretely expressing this abstract mutual exclusivity. The isolation world C3 is extended from No Form V, which in turn is extended from Z2, meaning that the three basic laws of formal logic are ultimately founded on the basis of form and no form. Although the evolution process of formal logic needs to use formal logic itself to express, this evolutionary mathematical structure itself gradually reveals formal logic.
In our evolution, the D3 version, the "true-false (or false-true)" version, and the traditional version of the three basic laws of formal logic become progressively more concrete. They evolved with "is" formal logic laws as their premise, because "is" and "is not" already appeared in C3. Another important aspect is that in this evolutionary process, we obtained the relationships between the identity of "is" ("A is A"), true-false, and "A is B".
However, this connects the core principles of formal logic (the three basic laws) with specific no form actions ("is" as manifestation and "negation" as motive force). This provides a deeper ontological foundation for these logical principles and avoids the infinite regression problem that arises when trying to base logic on other "logical" but equally unverifiable assertions.
Thus, we can clearly see that C3 representing A and FA representing non-A can transform into and oppose each other, while being unified at a higher level in D3. This is the concrete process of isolation dialectical logic. It views group evolution as a dialectical process of opposition and unity, thereby mapping a complex philosophical idea onto a rigorous and precise mathematical structure. This mathematical model not only explains abstract philosophical thoughts but also ensures logical consistency through the rigor of mathematical structures. This goes beyond analogy, showing that dialectical thinking is actually encoded in mathematical groups. The operations in C3 (a·a=b, b·b=a, a·b=c) are the most abstract dialectical processes, describing the most primitive relationships of opposition, interaction, and evolution between things. On this basis, only by expanding C3 into D3 did concrete dialectical processes evolve.
We see that when using C3 and FA to explain formal logic, when we say "A is A" in C3, then in FA it is "A is not non-A". This actually does not break A's identity, as it is still expressed from A's perspective - this is a characteristic of formal logic. If we express it from a different angle, similarly when we say "A is A" in C3, then in FA it becomes "non-A is not A". This is a symmetrical expression, but it negates A and thus breaks A's identity. When identity is broken, it must be rebuilt at a higher level, and rebuilding such identity means unifying in D3. This is the characteristic of isolation dialectical logic. The characteristics of these two types of logic have already been discussed in the previous section "Dialectical Logic".
Through the evolution from Z2 to No Form V to C3, and then to D3, we evolved the mathematical structure of formal logic. Thus, through the development from group Z2 to group D3, we evolved both dialectical logic and formal logic. They are both constructed on this mathematical model developed from the most basic Z2, and are closely connected. This mathematical model shows that no form action theory is not just a philosophical framework, but also a system theory that can be rigorously derived through mathematics. Through the mathematical evolution from Z2 to D3, no form action theory has transcended pure philosophical speculation to become a theoretical framework that can precisely describe logical and dynamic processes. This theoretical model has leaped from pure philosophical speculation to a method with potential for generating new insights and testable predictions. This theoretical model is not only a philosophical breakthrough but also a completely new method for constructing mathematics and logical science. This mathematical structure established for philosophy demonstrates how complexity emerges from the interactions of the simplest components.
We can combine field VFc with No Form V×V. Since No Form V×V can be extended into a ring, which is isomorphic to F4×F4, it can also be isomorphic to VFc×VFc. We call this ring extended from No Form V×V as VVRc (where R refers to ring) = VFc×VFc. Therefore, the addition operation in VVRc is (x,y)+(m,n)=(x+m,y+n), and the multiplication operation is (x,y)·(m,n)=(x·m,y·n), where the component operations in addition and multiplication come from VFc's addition and multiplication operations respectively. (e,e) is the additive identity element of VVRc, while (c,c) is the multiplicative identity element of VVRc.
This way we can use both VFc and No Form V×V operations in VVRc, thus combining them together. For example, adding identity (a,c) and distinction (c,a) results in being-for-itself (b,b), while multiplying them results in self (a,a). Multiplying identity (a,c) and distinction (c,a) is equivalent to ignoring the isolation form in both identity (a,c) and distinction (c,a) (because multiplying a and c in the components is equivalent to ignoring isolation c), thus becoming self. From this perspective, self is identity and distinction with isolation form ignored: self is self, but self must also make distinctions about itself.
4) Actually, the dihedral group D2 also has a reflection action like f in D3. Let's examine No Form V={e,a,b,c} which is isomorphic to D2. Since any of a,b,c can serve as the reflection action f, let's choose b as f. Thus, No Form V's C2={e,a}, FA={b,c} (where c=a·b), and through b's reflection action, C2 (which is Z2) and FA can transform into each other. For b's reflection action, if a and c are opposites (for example, identity and distinction), then this is a unity of opposites in isolation dialectical logic. If a and c are not opposites, then transformation through b's reflection is the usual no form united transformation. Moreover, any of a,b,c can serve as the reflection action f, and we can choose whichever we need as f based on practical requirements. This reflection action in No Form V is a useful method that can be used to find which concept unifies two opposites.
Next, let's use this method to find which concept unifies identity (a,c) and distinction (c,a):
Since identity (a,c), being-for-itself (b,b), and distinction (c,a) can constitute a no form integrated transformation, V_1={(e,e),(a,c),(b,b),(c,a)} is isomorphic to No Form V (meaning V_1 is a Klein four-group). Since (a,c) views manifestation from isolation, (a,c) is dominated by manifestation action, while (c,a) views isolation from manifestation, so (c,a) is dominated by isolation action. And (e,e) can be seen as pure no form. Therefore, (e,e) can be viewed as e, (a,c) as a, (b,b) as b, and (c,a) as c. We choose (b,b) as reflection f, thus the direct product V_1×V_1={(e,e),(a,c),(b,b),(c,a)}×{(e,e),(a,c),(b,b),(c,a)}, so "being (e,c)" in V_1×V_1 becomes ((e,e),(c,a)).
The above steps can continue to iterate. We find that the ground, which initially is (e,c) (that is, being, whose ground is itself), then becomes (((e,e),(e,e),...),((c,a),(a,c),...)), written simply as: ([e],[c,a]), representing concrete ground. We see that the initial ground only contains abstract isolation action c, gradually including identity (a,c) and distinction (c,a), as well as their more complex composite structures, meaning identity (a,c) and distinction (c,a) as opposing sides are unified in the ground. This way we have clarified the dialectical unity of identity (a,c) and distinction (c,a).
This dialectical unity obtained through mathematical methods is consistent with the explanation of "identity (a,c) and distinction (c,a) unified in ground" obtained through conceptual analysis in the section "Dialectical Logic". However, the dialectical unity obtained through mathematical methods is clearer and more detailed. This is the first time that an instance of isolation dialectical logic has been obtained through mathematical operations. This is sufficient to demonstrate the rationality of no form action theory.
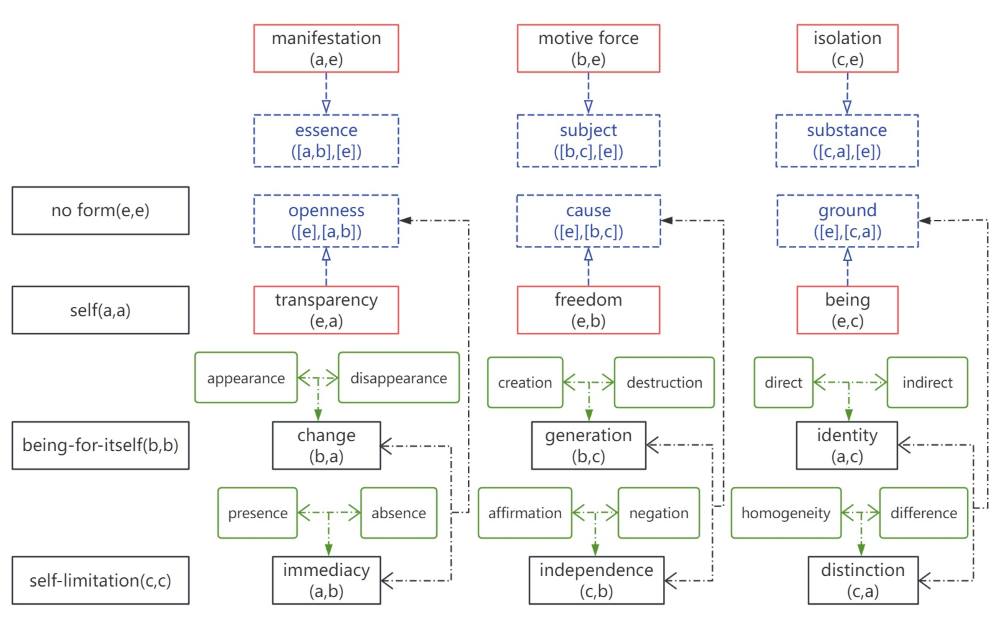
Thus, (e,c) as the most abstract ground is actually being, while concrete grounds contain identity and distinction at certain levels, and can be written as: ground ([e],[c,a]). Therefore, such a limit representation of being like (e,c) is the most abstract ground. This mathematical derivation aligns with my previous analysis of the relationship between ground and being: starting from one thing and continuously obtaining higher levels of ground in a limit way, the final limit is being, thus obtaining being as the ultimate ground, with being's ground being itself. For example, starting from thing a, a's ground is b, b's ground is c..., the final limit is being. Similarly, transparency (e,a) is the most abstract openness, freedom (e,b) is the most abstract cause, manifestation (a,e) is the most abstract essence, motive force (b,e) is the most abstract subject, isolation (c,e) is the most abstract substance.
Similarly, generation (b,c), self (a,a), and independence (c,b) can constitute no form integrated transformation. Cause (e,b) becomes (((e,e),(e,e),...),((b,c),(c,b)...)). Through continuous iteration, we obtain: generation (b,c) and independence (c,b) are unified in cause. Concrete cause can be written as: cause ([e],[b,c]).
Similarly, change (b,a), self-limitation (c,c), and immediacy (a,b) can constitute no form integrated transformation. Openness (e,a) becomes (((e,e),(e,e),...),((a,b),(b,a)...)). Through continuous iteration, we obtain: change (b,a) and immediacy (a,b) are unified in openness. Concrete openness can be written as: openness ([e],[a,b]).
Since manifestation (a,e) and transparency (e,a) are in opposite directions, essence (a,e) becomes (((a,b),(b,a),...),((e,e),(e,e)...)). Therefore, change (b,a) and immediacy (a,b) are unified in essence in an opposite way. Concrete essence can be written as: essence ([a,b],[e]).
Similarly, generation (b,c) and independence (c,b) are unified in subject in an opposite way. Concrete subject can be written as: subject ([b,c],[e]).
Similarly, identity (a,c) and distinction (c,a) are unified in substance in an opposite way. Concrete substance can be written as: substance ([c,a],[e]).
Moreover, we can also iterate [c,a] into independence (c,b), becoming ([c,a],[b]). However, there is a slight difference here, which is that there is motive force b in this combination. Since independence (c,b) views isolation from the perspective of motive force, the question becomes viewing the isolation in identity (a,c) and the isolation in distinction (c,a) from the perspective of motive force. Thus, viewing the isolation in identity (a,c) from the perspective of motive force becomes affirmation (that is, "A is B"), while viewing the isolation in distinction (c,a) from the perspective of motive force becomes negation. Moreover, affirmation and negation as opposing sides are unified in independence. Through this combination, we clearly see that both "affirmation" and "negation" contain motive force b. In other words, in the world of isolation, there are two types of motive force: affirmation and negation.
Similarly, we can obtain:
(1) Iterating [a,c] into immediacy (a,b), becoming ([a,c],[b]). Thus obtaining: presence and absence as opposing sides are unified in immediacy (a,b). Note: ([a,c],[b]) and ([c,a],[b]) are different - ([a,c],[b]) views manifestation from the perspective of motive force, while ([c,a],[b]) views isolation from the perspective of motive force.
(2) Iterating [b,c] into change (b,a), becoming ([b,c],[a]). Thus obtaining: appearance and disappearance as opposing sides are unified in change (b,a).
(3) Iterating [a,b] into identity (a,c), becoming ([a,b],[c]). Thus obtaining: direct and indirect as opposing sides are unified in identity (a,c).
(4) Iterating [c,b] into distinction (c,a), becoming ([c,b],[a]). Thus obtaining: homogeneity and difference as opposing sides are unified in distinction (c,a).
(5) Iterating [b,a] into generation (b,c), becoming ([b,a],[c]). Thus obtaining: creation and destruction as opposing sides are unified in generation (b,c).
(6) Iterating [c,a] into independence (c,b), becoming ([c,a],[b]). Thus obtaining: affirmation and negation as opposing sides are unified in independence (c,b). This has already been discussed.
We discover that two opposing concepts can be iterated into different concepts in different ways, and will produce different results of dialectical unity.
Through iteration, we discover that in these six groups, originally two-dimensional elements become three-dimensional elements - for example, (c,b) becomes ([c,a],[b]). Through this iteration, non-trinity elements become no form trinity elements.
These have already been explained through conceptual analysis in the section "Dialectical Logic". However, using mathematical derivation now makes the opposition and unity between these concepts clearer and more precise. As shown in the figure: (where black and green arrows represent the opposition and unity between concepts, blue arrows represent the limit process.)
Until now, I have constructed the core content of my developed no form action theory framework on mathematical structures.
The above iteration of the Klein four-group is a powerful method. As mentioned before, there are 35 Klein four-groups in the subgroups of No Form V×V, and iterating each of them will yield different results. Moreover, they can undergo cross-iteration (for example, first iterating with {(e,e), (e,a), (b,b), (b,c)}, then with {(e,e), (e,a), (c,e), (c,a)}), which will produce very rich results. Through this method, we can precisely obtain the relationships between different concepts, while also seeing how these concepts combine precisely in a mathematical way.
5) Demonstration of the Relationship Between Philosophy and Mathematics
The continuous expansion starting from Z2 (as no form and form) demonstrates the process of no form constantly transforming into form. Even if we first disregard the mathematical structures within it, this transformation process must necessarily be one where form continuously strengthens and structure becomes further clarified, so this transformation process must necessarily be accompanied by a pure formalized structure. And mathematics is the purest formalized structure, therefore, this transformation process must necessarily possess such a pure formalized mathematical structure. Mathematical form is not merely a descriptive tool, but an ontological necessity for understanding and expressing the transformation from "no form" to "form".
Our rational understanding of this world is actually an understanding of isolation form, and the purest understanding is mathematical understanding, therefore, the mathematization of philosophy is inevitable.